

Sponsored Links
TOP > Techniques > Hidden Unique Rect's.
Solving Techniques 17
Hidden Unique Rect's.
This is a method of finding hidden unique rectangles.
Below, the [6] in the [156] is X’d out. It can be eliminated as a candidate.
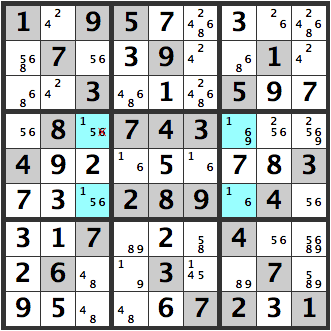
If the upper left blue [156] cell is a [1], then [6] can’t be a candidate for this cell.
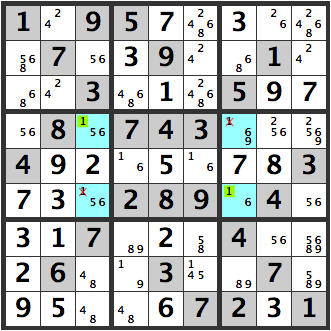
A problem arises when the blue [156] is any number other than [1]. The yellow parts become candidates for [1].
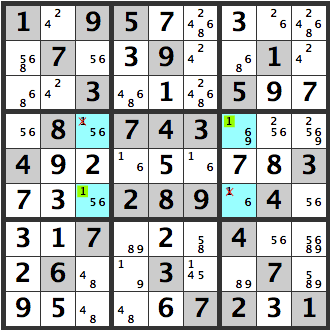
Then the lower right blue cell would be a [6], since it is a [16]. In this case, too, if the upper left, blue cell is a [6]…
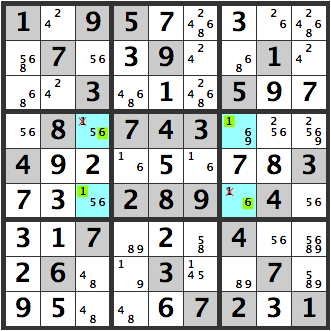
we eventually arrive at the following diagram.
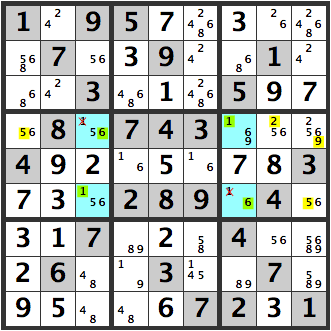
But lets step back. The four blue cells create a unique rectangle with [16][16], leading to multiple solutions. Therefore, in this case, the blue cell to the upper left is a [5] and not a [6].
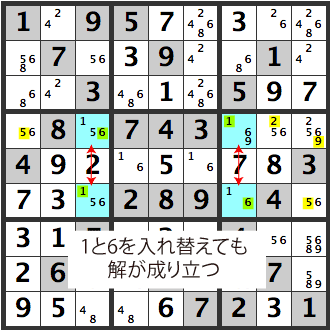
Hence, this [6] can be removed as a candidate. Otherwise, we are left with a Sudoku problem which doesn’t work.
Sponsored Links
Hidden Unique Rect's. 2
Below is also a hidden unique rectangle. The X’d out [6] can be removed as a candidate from [R4C3]. (The 8s in the same row form a strong link)
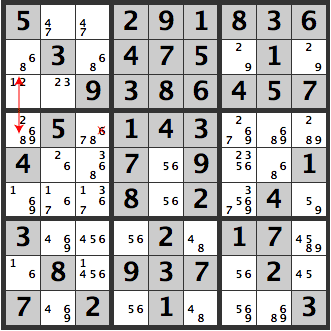
If the [68] in the upper left [R2C1] is an [8], then [R2C3] becomes a [6], so the X’d out [6] in [R4C3] can’t be entered there.
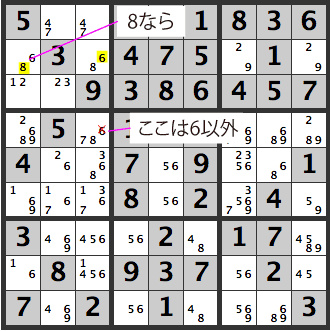
If the upper left [R2C1]’s [68] is a [6], we have a problem. In this case, there are only two [8]’s in the same row, so even if there are many candidates for [R4C1], it will be an [8]. Furthermore, [R2C3] will also be an [8]. If [R4C3] is a [6], we have a unique triangle where [6] and [8] are interchangeable.
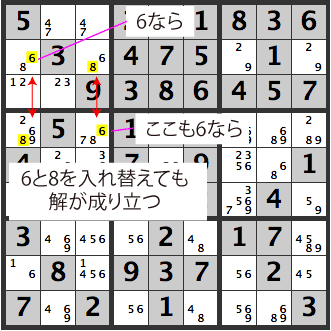
It will hence, not work as a Sudoku problem, and [6] can be removed as a candidate.
Names of cells in Sudoku
| R1C1 | R1C2 | R1C3 | R1C4 | R1C5 | R1C6 | R1C7 | R1C8 | R1C9 |
| R2C1 | R2C2 | R2C3 | R2C4 | R2C5 | R2C6 | R2C7 | R2C8 | R2C9 |
| R3C1 | R3C2 | R3C3 | R3C4 | R3C5 | R3C6 | R3C7 | R3C8 | R3C9 |
| R4C1 | R4C2 | R4C3 | R4C4 | R4C5 | R4C6 | R4C7 | R4C8 | R4C9 |
| R5C1 | R5C2 | R5C3 | R5C4 | R5C5 | R5C6 | R5C7 | R5C8 | R5C9 |
| R6C1 | R6C2 | R6C3 | R6C4 | R6C5 | R6C6 | R6C7 | R6C8 | R6C9 |
| R7C1 | R7C2 | R7C3 | R7C4 | R7C5 | R7C6 | R7C7 | R7C8 | R7C9 |
| R8C1 | R8C2 | R8C3 | R8C4 | R8C5 | R8C6 | R8C7 | R8C8 | R8C9 |
| R9C1 | R9C2 | R9C3 | R9C4 | R9C5 | R9C6 | R9C7 | R9C8 | R9C9 |
Sponsored Links


