Sponsored Links

TOP > Techniques > Sword-Fish

Solving Techniques 9
Sword-Fish

This is a situation where the 1 is only possible in three places, like columns 2, 5 and 8, below. (there are only three cells where 1 can be, in the columns indicated with red lines, and they occur in the same rows)

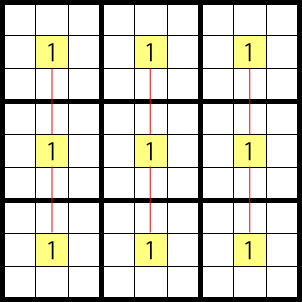
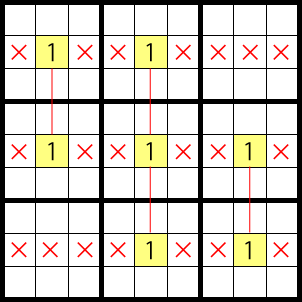
In this case, the 1’s can’t be in the places marked with x’s as pictured below, so they can be eliminated as candidates.

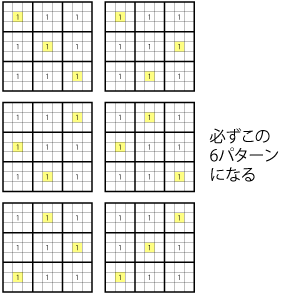
When examined, you see that there are only six ways in which the 1 can be entered, and that indeed, they can’t be entered where the x’s are.

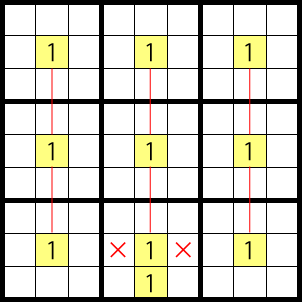
As a variation there is a pattern like the one below.

It’s a very efficient technique when this pattern actually happens, since you can eliminate lots of candidates at once.

Finned Sword-Fish

As in the diagram below, when there are many candidates for 1 in the middle column only, it is called a finned sword fish. The cells indicated with x only, can be eliminated as candidates for 1. (also possible when the pattern is not in the center)

Lets examine if this is true. As shown below, if there is a 1 in the ‘fin’ area, there can’t be another 1, other than in this box. However, in column 2 and column 8, there are three places each for where 1 could be. Therefore, the possibilities can’t be eliminated the same way as they can, when there are no fins.

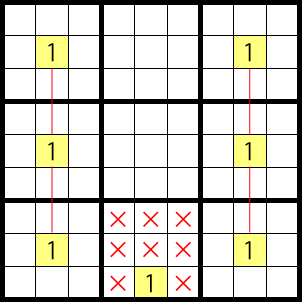
As shown below, if a part that is not the fin becomes a 1, then a 1 can’t be in 3 rows, just as it would, when there are no fins.

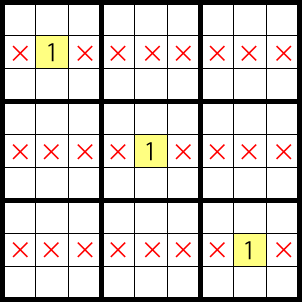
Therefore, the candidates that can be eliminated based on the overlap, are the cells that would get eliminated in a swordfish, but only within the box, which the fin is located in.

The swordfish is quite challenging, but when examined, it does hold up to be true.

Names of cells in Sudoku

R1C1	R1C2	R1C3	R1C4	R1C5	R1C6	R1C7	R1C8	R1C9
R2C1	R2C2	R2C3	R2C4	R2C5	R2C6	R2C7	R2C8	R2C9
R3C1	R3C2	R3C3	R3C4	R3C5	R3C6	R3C7	R3C8	R3C9
R4C1	R4C2	R4C3	R4C4	R4C5	R4C6	R4C7	R4C8	R4C9
R5C1	R5C2	R5C3	R5C4	R5C5	R5C6	R5C7	R5C8	R5C9
R6C1	R6C2	R6C3	R6C4	R6C5	R6C6	R6C7	R6C8	R6C9
R7C1	R7C2	R7C3	R7C4	R7C5	R7C6	R7C7	R7C8	R7C9
R8C1	R8C2	R8C3	R8C4	R8C5	R8C6	R8C7	R8C8	R8C9
R9C1	R9C2	R9C3	R9C4	R9C5	R9C6	R9C7	R9C8	R9C9

• TOP
• My page(Login)
• Daily Sudoku
• Back Numbers
• Sudoku puzzles
• LEVEL:1 ->LEVEL:2
• LEVEL:3 ->LEVEL:4
• LEVEL:5 ->LEVEL:6
• LEVEL:7 ->LEVEL:8
• Sai(彩) mode Sudoku puzzles
• LEVEL:1 ->LEVEL:2
• LEVEL:3 ->LEVEL:4
• LEVEL:5 ->LEVEL:6
• LEVEL:7
• Sudoku 16
• LEVEL:1 ->LEVEL:2
• LEVEL:3 ->LEVEL:4
• LEVEL:5 ->LEVEL:6
• LEVEL:7
• Sudoku 6
• LEVEL:1 ->LEVEL:2
• LEVEL:3 ->LEVEL:4
• LEVEL:5
• Average time
• Top 300 difficult puzzles
• High Score
• Medals
• Rules
• Sudoku Tricks
• Solving Techniques
• Hidden Singles
• Naked Pairs/Triples/Quads
• Hidden Pairs/Triples/Quads
• Pointing Pairs
• X-Wing
• Y-Wing
• Simple Coloring
• Simple Chain
• Sword-Fish
• XYZ-Wing
• X-Cycles
• BUG+1
• XY-Chains
• Jelly Fish
• Unique Rectangles
• Extended Unique Rect.
• Hidden Unique Rect's
• WXYZ-Wing
• Aligned Pair Exclusion
• Sudoku problem solver program
• How to create Sudoku problems
• 1 Creating the answer
• 2 Creating blank boxes
• 3 Sudoku solver program
• 4 Creating problems with fewer clues
• About Sudoku (column)
• About Sudoku
• Possible combinations of Sudoku
• About Sudoku techniques
• Contact
• Download