Sudoku Kyo free sudoku game

Sponsored Links

TOP > Techniques > Pointing Pairs

Solving Techniques 4
Pointing Pairs

It often happens that the possibilities of boxes, rows and columns have been exhausted and eight cells are filled with two remaining (the technique also works with three remaining). Also, there are cases where the two remaining cells are next to each other either in a row or column. This technique can be applied in these cases.

Pointing Pairs

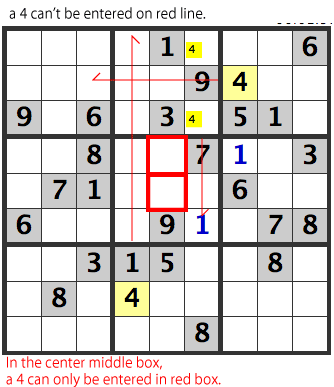
Let’s look at the upper middle box. There is a 4 in [R8C4], so it can’t be entered in the 4th column.
Also, since there is a 4 in the second row, [R2C7], a 4 can’t be entered there.
Hence, the cells where a 4 can be entered in the upper middle box, are either [R1C6] or [R3C6].
Therefore, in the center middle box, a 4 can only be entered in column 5.
As you can see, in pointing pairs, you look for pairs or triple numbers and eliminate the possibility of them belonging in other cells.

Sponsored Links

Pointing Pairs-2

In the upper middle box, a 6 can only be entered in row [2].
Similarly, in the center middle box, a 6 can only be entered in row [4].

Now that we have a [6,6] pair in the upper middle box, a 6 can’t be entered in the lower middle box’s columns 4 and 5.

Hence, in the lower middle box, a 6 is only possible in column 6.
As you can see, this is a technique of slowly narrowing down the candidate numbers.

Names of cells in Sudoku

R1C1R1C2R1C3R1C4R1C5R1C6R1C7R1C8R1C9
R2C1R2C2R2C3R2C4R2C5R2C6R2C7R2C8R2C9
R3C1R3C2R3C3R3C4R3C5R3C6R3C7R3C8R3C9
R4C1R4C2R4C3R4C4R4C5R4C6R4C7R4C8R4C9
R5C1R5C2R5C3R5C4R5C5R5C6R5C7R5C8R5C9
R6C1R6C2R6C3R6C4R6C5R6C6R6C7R6C8R6C9
R7C1R7C2R7C3R7C4R7C5R7C6R7C7R7C8R7C9
R8C1R8C2R8C3R8C4R8C5R8C6R8C7R8C8R8C9
R9C1R9C2R9C3R9C4R9C5R9C6R9C7R9C8R9C9

Sponsored Links

Sudoku Kyoto（Number Place）Free Puzzle Game
TOP